Imagine you’re standing on a busy street, watching cars zoom by. Have you ever noticed that the lines painted on the road, the ones that guide traffic, seem to run perfectly straight and never cross each other? They’re like two long, unwavering friends, always keeping their distance. These lines represent parallel lines, a concept that plays a pivotal role in geometry and is the foundation for understanding a variety of real-world applications, from architecture and engineering to art and design. But what happens when a third line, called a transversal, cuts through these parallel lines? It’s at this point that fascinating relationships emerge and a world of angles opens up, one that can be both exciting and challenging – especially for students in the crucial 2-7 grade range where geometric reasoning is developing.

Image: www.coursehero.com
This guide is designed to help students in grades 2-7 navigate the world of parallel lines and transversals, offering a comprehensive exploration of the key concepts and providing real-world connections to make learning engaging and relatable. We’ll unlock the secrets of angles, delve into the special relationships formed by parallel lines and transversals, and equip students with the tools to conquer any geometric problem with confidence.
Parallel Lines and Their Undying Friendship
Parallel lines are like two best friends, walking side-by-side, always maintaining the same distance from each other. They never cross paths, no matter how far they extend. This constant distance is the key to understanding parallel lines: they have the same slope or direction. Think of it like this: if you were to draw a line connecting any two points on one parallel line and another line connecting two points on the other parallel line, these lines would always be parallel to each other, never meeting at a point.
Imagine two long, straight roads running parallel to each other in your neighborhood. They never intersect, just like parallel lines in geometry. This simple analogy helps visualize the concept of parallel lines and makes it easier to grasp.
The Transversal: A Line That Breaks the Pattern
Now, let’s introduce a new player: the transversal. This line, like a curious explorer, cuts through the parallel lines. Imagine a crossroad intersecting those parallel roads in your neighborhood. That crossroad acts as a transversal, cutting through the existing parallel lines. It’s a seemingly simple addition, yet it dramatically changes the picture by forming unique relationships between the parallel lines.
Angles Galore: Exploring the Relationships
When a transversal intersects parallel lines, it creates various angles. These angles have special relationships with each other, making them easier to understand and calculate. Let’s break down some key terms:
-
Corresponding Angles: Imagine you’re driving down one of the parallel roads. As you approach the transversal, you’ll see an angle on your left side. Now, picture yourself driving down the other parallel road, approaching the same transversal. The angle you see on your left side will be identical to the one on the left side of the first road. These are called corresponding angles, located in the same position relative to the transversal and parallel lines. They’re like twins, always equal in measure.
-
Alternate Interior Angles: Let’s switch gears and focus on the angles formed inside the parallel lines. Notice two angles located on opposite sides of the transversal, within the space created by the parallel lines? They are called alternate interior angles and, like good friends, they have equal measures.
-
Alternate Exterior Angles: Now, let’s move to the outside of the parallel lines. Two angles located on opposite sides of the transversal, outside of the parallel lines, are called alternate exterior angles. Similar to the alternate interior angles, they are also equal in measure.
-
Same-Side Interior Angles: These angles are located inside the parallel lines on the same side of the transversal. Unlike the other pairs, they are not equal. They are supplementary angles, meaning they add up to 180 degrees.
Bringing it all Together: Real-World Applications
Parallel lines and transversals are not just abstract concepts confined to textbooks. They hold immense value in understanding real-world situations. Here are a few examples:
-
Architecture and Construction: Architects use parallel lines to ensure buildings are structurally sound, creating walls, floors, and ceilings that run parallel to each other. Transversals come into play when designing roof structures, ensuring stability and proper support.
-
Engineering and Design: Engineers rely on the principles of parallel lines and transversals when designing bridges, roads, and other infrastructure. Understanding the relationships between angles helps in constructing secure and resilient structures.
-
Art and Design: Artists utilize the concepts of parallel lines and transversals to create depth, perspective, and visual harmony in their creations. Think of the lines of a cityscape converging in the distance, creating a sense of scale and realism – a perfect embodiment of parallel lines meeting at a vanishing point.
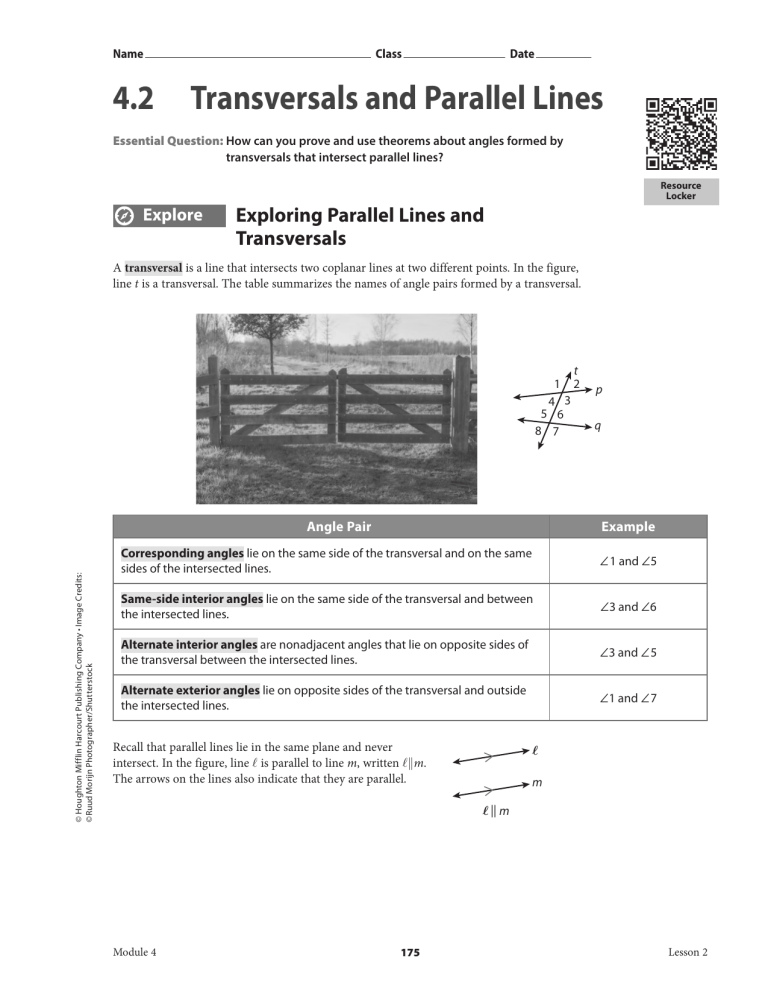
Image: lamborghini-islero.com
Mastering the Concepts: A Step-by-Step Approach
Now that we’ve explored the key concepts and real-world applications of parallel lines and transversals, let’s provide a practical guide to help students grasp these ideas:
-
Visualization is Key: Encourage students to visually represent parallel lines and transversals by drawing them on paper or using real-world objects like popsicle sticks or chopsticks. This hands-on approach fosters deeper understanding and reinforces the concepts.
-
Angle Measuring Tools: Provide students with protractors to measure the angles formed when a transversal intersects parallel lines. This provides tangible evidence of the relationships between angles, demonstrating visually that corresponding angles, alternate interior angles, and alternate exterior angles are indeed equal in measure.
-
Interactive Learning: Utilize online resources and interactive games to enhance the learning experience. These platforms provide engaging visuals and interactive challenges to make the learning process more fun and stimulating.
-
Real-World Examples: Connect the learning to students’ everyday experiences. Point out the applications of parallel lines and transversals in their environment – the stripes on a zebra crossing, the lines on a football field, or the rails of a train track.
-
Practice, Practice, Practice: Consistent practice is crucial for mastery. Encourage students to solve practice problems, focusing on identifying corresponding, alternate interior, and alternate exterior angles, as well as using their knowledge to calculate unknown angles.
2 7 Study Guide And Intervention Parallel Lines And Transversals
https://youtube.com/watch?v=vYvVsYjr2Ec
Empowering Students with the Tools to Succeed
Learning about parallel lines and transversals is not just about memorizing definitions and theorems. It’s about developing logical thinking skills, spatial reasoning, and a deeper understanding of the world around us. By providing students with a comprehensive guide, engaging visuals, and real-world examples, we can empower them to excel in geometry and confidently navigate the exciting world of shapes and angles.






